Which Form 8865 Reduction 2023-2024

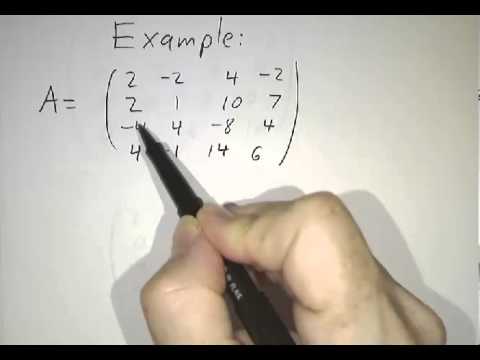
In this video, we're going to review the machinery of row reduction and how we turn a general matrix into a matrix in row echelon form, which is good, and reduced row echelon form, which is best. The rules of the game are that if somebody gives you a matrix, a row operation is something that you do where you can rescale a row, which means multiplying a row by any nonzero constant, or you can add a multiple of one row to another row, or you can swap two rows. These are called row operations, and the goal is if somebody gives you a matrix, you want to apply a bunch of row operations until the matrix either becomes in row echelon form or reduced row echelon form. So, what row echelon form means is that some of the rows might be nothing but zeros. If you have a row that is nothing but zeros, it has to be at the very bottom of the matrix. Every other row has a first nonzero entry. The first nonzero entry is called a pivot. So, this is a pivot, this is a pivot, this is a pivot, this is a pivot. It's the first non-zero entry of a row. Row echelon form means that each pivot is to the right of the previous pivot. So, you have the staircase, it escalates cyclis French for stairs, that you go to the right till you get to the pivot of the next row, then you go to the right till you get to the pivot of the next row, go to the right. Now, I drew it where it's one to the right, you could also have something where you go two to the right or three to the right or 4 to the...